Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Physical Address
304 North Cardinal St.
Dorchester Center, MA 02124
Contents
The circumference of a circle is a fundamental concept in geometry, representing the total length of the boundary of the circle. It is crucial for various real-world applications, such as calculating the distance around circular objects like wheels, plates, or even celestial bodies. In this article, we will delve into the definition of the circumference of a circle, explore the formula to calculate it, understand the relationship between circumference and diameter, and address common FAQs related to this topic.
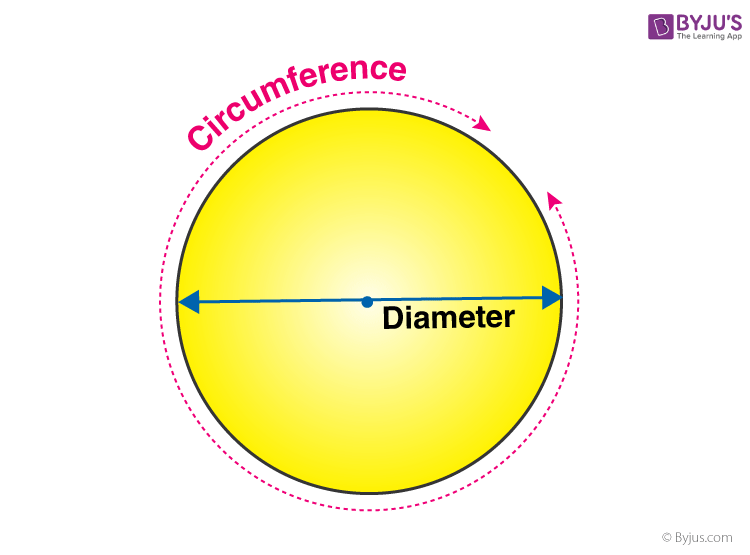
The circumference of a circle is defined as the perimeter or the boundary of the circle. It is the total length of the outer edge of the circle, similar to how we measure the perimeter of polygons. The circumference is a linear measurement and is expressed in units of length such as centimeters, meters, or kilometers.
The formula to calculate the circumference of a circle involves the circle’s radius ‘r’ and the mathematical constant π (pi). The formula is given as Circumference = 2πr, where ‘r’ is the radius of the circle. Alternatively, the circumference can also be calculated using the diameter with the formula Circumference = π × Diameter. This relationship between circumference, radius, and diameter is essential in circle geometry.
To find the circumference of a circle, you can use the radius or diameter along with the value of π. For example, if the radius of a circle is 25 units, you can substitute this value into the circumference formula (C = 2πr) to determine the total length of the circle’s boundary. Understanding how to calculate circumference is vital for various mathematical and practical applications.
The relationship between circumference and diameter is defined by the constant π. When the diameter of a circle is known, the circumference can be calculated using the formula C = πd, where ‘d’ represents the diameter. This relationship highlights the significance of π in connecting the key metrics of a circle and is a fundamental concept in mathematics and physics.
There are several common questions related to the circumference of a circle, such as how to find the circumference from the diameter, how to calculate the area of a circle using circumference, and the units used to measure circumference. Understanding these FAQs provides a comprehensive insight into the concept of circumference and its practical implications.
Overall, the circumference of a circle plays a crucial role in geometry, mathematics, and various real-world scenarios. By grasping the fundamentals of circumference calculation and its relationship with other circle parameters, individuals can enhance their problem-solving skills and mathematical proficiency.